On Measure Transformed Canonical Correlation Analysis
Dr. Koby Todros, University of Michigan
2:00pm Wednesday, 2 May 2012, ITE 325b
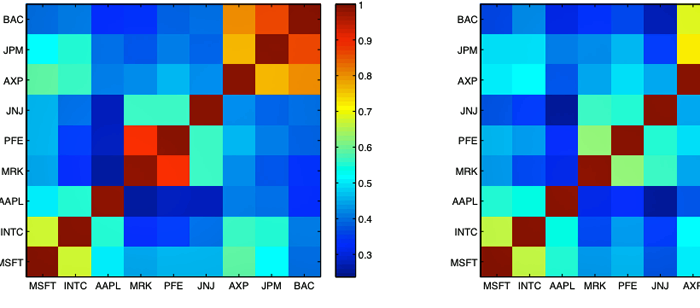
In this work linear canonical correlation analysis (LCCA) is generalized by applying a structured transform to the joint probability distribution of the considered pair of random vectors, i.e., a transformation of the joint probability measure defined on their joint observation space. This framework, called measure transformed canonical correlation analysis (MTCCA), applies LCCA to the data after transformation of the joint probability measure. We show that judicious choice of the transform leads to a modified canonical correlation analysis, which, in contrast to LCCA, is capable of detecting non-linear relationships between the considered pair of random vectors. Unlike kernel canonical correlation analysis, where the transformation is applied to the random vectors, in MTCCA the transformation is applied to their joint probability distribution. This results in performance advantages and reduced implementation complexity. The proposed approach is illustrated for graphical model selection in simulated data having non-linear dependencies, and for measuring long-term associations between companies traded in the NASDAQ and NYSE stock markets.
Koby Todros was born in Ashkelon, Israel, in 1974. He received his B.Sc., M.Sc., and Ph.D. degrees in electrical engineering at 2000, 2006, and 2011, respectively, from the Ben-Gurion University of the Negev. He is currently a post-doctoral fellow with the Department of Electrical Engineering and Computer Science, in the University of Michigan. His research interests include statistical signal processing and estimation theory with focus on association analysis, uniformly optimal estimation in the non-Bayesian theory, performance bounds for parameter estimation, blind source separation, and biomedical signal processing.